Welcome to The Centre for Complex Systems

The centre is one of the largest in the UK working on statistical mechanics aspects of dynamical systems and stochastic systems, and on complex systems and complex networks.

We have a broad range of research interests in mathematical foundations of dynamical systems theory, in statistical physics and stochastic modelling methods as applied to non-equilibrium situations, and in the mathematical description and modelling of the architecture and dynamics of complex systems and networks. A significant part of our research work has applications in an interdisciplinary context.

Our theoretical research explores cutting-edge topics in

• Non-equilibrium processes
• Stochastic modelling
• Ergodic theory
• Topological dynamics     
• Nonlinear dynamics and chaos
• Network theory

Applied research highlights include

• Cancer evolution and species interactions in biological systems
• Mathematical and Digital Epidemiology
• Social networks, team dynamics and success
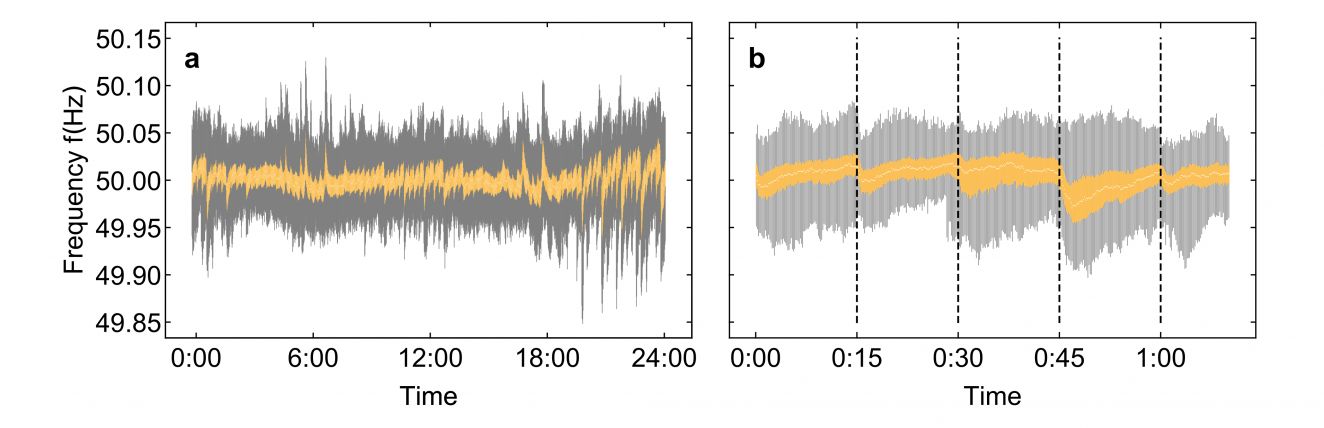
• Quantitative urbanism, smart cities and energy
• Sustainable energy systems and consumer behaviour
• Statistical aspects of air pollution and water quality
• Movement Ecology

The research outputs of our Centre for Complex Systems are highly cited: In total the published work of the academic staff members in our centre has received more than 100,000 citations. The centre has an excellent track record in publishing in high impact journals: in the past 5 years we have published articles in Nature, Nature Communications, Nature Physics, Nature Machine Intelligence, Nature Human Behaviour, Nature Energy, Nature Genetics, Science Advances, and many more top journals.